The “box” or tabular method of multiplication is a great way for elementary, middle, and high school students to make mathematical connections. In this first of two articles, I discuss how the box method can be used to multiply and divide numbers.
The next article explains how the box method can be extended to polynomials.
NOTE: This article expands on ideas presented at a Math for America workshop that I co-facilitated in 2017 in New York City.
Basic principles
The basic principle behind the box method can be illustrated with the problem below:
1. In rectangle ABCD, AB = 5, BF = 2, and FC = 3. Find the areas of ABFE, EFCD, and ABCD. What is the relationship between these areas?
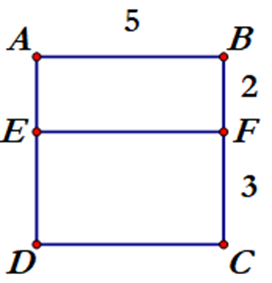
The area of ABCD is AB(BC) = 5(5) = 25. The area of ABCD is also the combined areas of ABFE and EFCD, or 5(2) + 5(3). Thus, 5(5) = 5(2) + 5(3).
Since the area of the large rectangle is equal to the combined areas of the smaller rectangles, then we can generalize #1 by saying m(x + y) = mx + my for any values of m, x, and y. Geometrically, the box method also illustrates the Distributive Property and the Partition Postulate (“the whole is equal to the sum of its parts”).
Multiplying numbers
The box method can be used to multiply whole numbers.
2. 32(21)
We could illustrate this problem with squares arranged in a 32 x 21 rectangle:
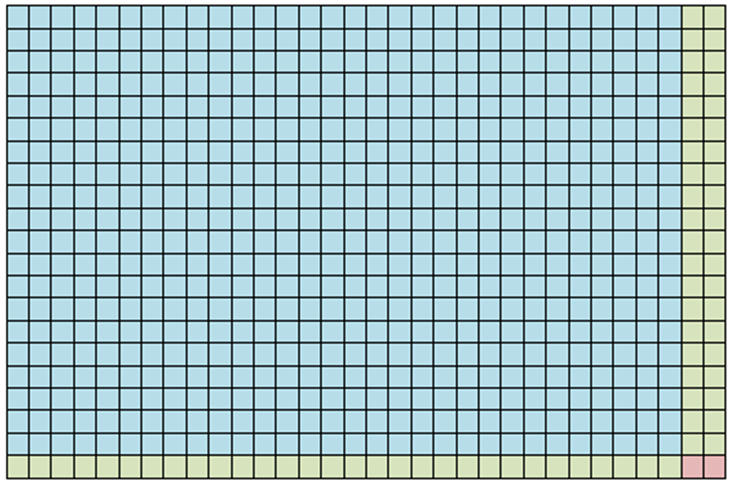
To multiply the numbers, we write each number in expanded form. In this case, 32 = 3(101) + 2(100) and 21 = 2(101) + 1(100). We construct a 2 x 2 rectangle and find the area of each smaller rectangle by multiplying its length and width, Then we find the total area by adding the areas of each of the smaller rectangles. The area of the entire rectangle equals the area of the smaller rectangles.
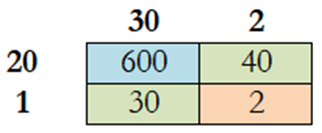
Note that the sum of the diagonals in the box correspond to the places in the product. The first diagonal (in blue) is the hundreds (600), the second (in green) is the tens (40 + 30 = 70), and the third (in orange) is the ones (2). The total area is 600 + (40 + 30) + 2 = 600 + 70 + 2 = 672.
The box method has the same calculations as the vertical method of multiplication, but the work is written differently:
32
x 21
32
+ 640
672
In my experience, I’ve found that students generally make fewer mistakes with the box method than with other methods because the numbers in the box method are lined up in a more organized way.
Note also that the dimensions of the rectangle could have been reversed, resulting in the same product. This nicely illustrates the commutative property of multiplication, i.e. ab = ba. We can understand this geometrically by noting that rotating a rectangle does not change its area.
3. (314)(123)
The box method can also be used to multiply larger numbers:
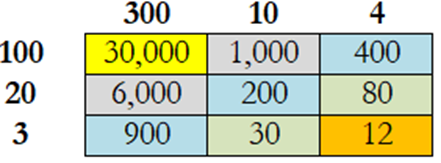
Adding diagonally gives 30,000 + (6,000 + 1,000) + (900 + 200 + 400) + (30 + 80) + 12 = 30,000 + 7,000 + 1,500 + 110 + 12 = 38,622. Adding the diagonals in a problem like this can get a little tricky since some of the diagonal sums that add up to more than 10 must be “carried over” into the place to the left. The “lattice” method of multiplication (explained on the Math Forum http://mathforum.org/library/drmath/view/59087.html) and the Exploding Dots method (explained by James Tanton at http://gdaymath.com/courses/exploding-dots/) provide other ways to handle this addition.
4. (2,230)(101)
When we multiply numbers with zeroes, we should add a 0 row or column as a placeholder. While not required, it reinforces the idea that the sum of the diagonals represent the places in the product.
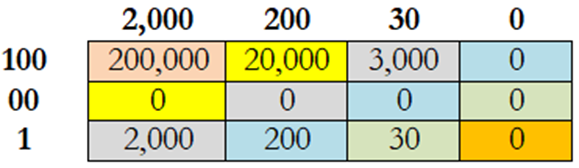
The sum is 200,000 + 20,000 + (3,000 + 2,000) + 200 + 30 = 200,000 + 20,000 + 5,000 + 200 + 30 = 225,230.
5. (0.125)(0.38)
The box method can also be used to multiply decimals, as long as each number being multiplied is separated into places:
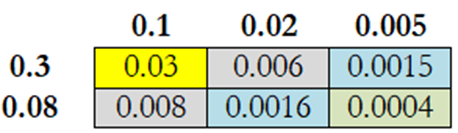
(0.125)(0.38) = 0.03 + (0.006 + 0.008) + (0.0015 + 0.0016) + 0.0004 = 0.0475
Dividing whole numbers
The box method easily relates multiplication and division. Multiplication using the box is finding the area of a rectangle given its dimensions. Division using the box method is the reverse of multiplication: given the area of a rectangle and one of its dimensions, find the other dimension.
6. 483 ÷ 21
We know from #2 above that we will need a 2 x 2 rectangle here. We know from #2 that since the dividend has three places, then the resulting rectangle must have three diagonals. We know that we must have either two rows or two columns in the rectangle since the divisor 21 has two places, so we can set up the following rectangle:

We know that the product, which is the sum of the diagonals, is the dividend 483, so we can represent it at the bottom of the diagram. I’ve found that writing each diagonal sum and drawing corresponding arrows from the diagonal helps students keep their work organized and helps graders follow their work.
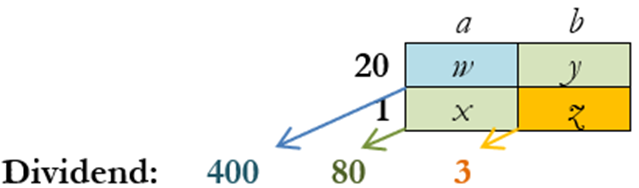
(To facilitate the explanation, I’ve included the variables a, b, w, x, y, and z in the diagram, but on student work I wouldn’t expect these.)
We know that 400 goes in the top left box (w), since there is only one box in the rectangle that represents the hundreds place of the dividend.

Now we work in reverse: what times 20 equals 400? (or, what is 400 ÷ 20?) We write the answer, 20, above the top left box (a). Next, we multiply 20(1) to get 20, which is the value of x:
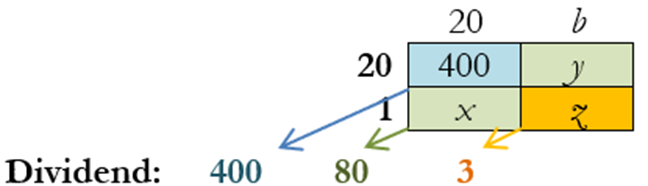
Since 20 + y = 80, then y = 80 – 20 = 60:
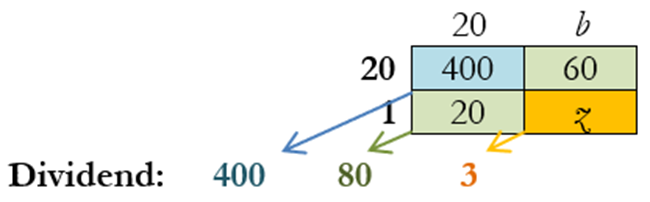
We divide 60 ÷ 20 = 3 = b and multiply 3(1) = 3 = z.
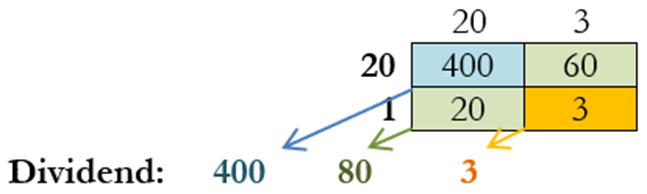
Thus, our answer is 23.
We can check it by multiplying 23(21) to see that the product is 400 + 80 + 3 = 483.
7. 488 ÷ 21
The work here is similar to that in the previous problem, except that there is a remainder of 5.
We can check this work by multiplying 23(21) and adding 5: 23(21) + 5 = 483 + 5 = 488.
Final Notes
For more information on using the box method to divide, see Karen Campe’s blog post at https://karendcampe.wordpress.com/2023/08/16/area-arrangements/. Howie Hua has videos explaining how to use the box method for division at https://www.youtube.com/watch?v=JcSLqinB3yA and https://www.youtube.com/watch?v=4ahm8BfQWMg.
The box method is a great way to relate algebraic and geometric representations of numbers. Students who master this method in elementary school can extend it to more abstract problems in middle and high school math, as I explain in the next part.